list of all geometry formulas pdf
A comprehensive list of geometry formulas in PDF format is a valuable resource for students, educators, and professionals․ These PDFs often include formulas for calculating area, perimeter, volume, surface area, and other geometric properties of various shapes, from basic two-dimensional figures like triangles and circles to more complex three-dimensional objects like cubes and spheres․ These resources can be particularly helpful for reviewing key concepts, preparing for exams, or solving practical problems that involve geometric calculations․
Introduction
Geometry, the study of shapes, sizes, and positions, plays a crucial role in various fields, including mathematics, engineering, architecture, and design․ Mastering the fundamental formulas of geometry is essential for understanding and solving problems related to these disciplines․ A comprehensive list of geometry formulas in PDF format serves as a valuable reference tool for students, educators, and professionals alike․ These PDFs typically encompass a wide range of formulas, covering basic shapes like triangles, squares, and circles, as well as more complex three-dimensional objects like cubes, spheres, and cylinders․ They provide a concise and organized compilation of essential equations, making it easier to recall and apply them in different contexts․
Basic Shapes
The foundation of geometry lies in understanding the properties and formulas associated with fundamental shapes․ These shapes, often categorized as two-dimensional (2D) and three-dimensional (3D), form the building blocks for more complex geometric concepts․ 2D shapes, such as triangles, squares, rectangles, and circles, exist on a flat plane and are defined by their perimeter and area․ 3D shapes, on the other hand, have volume and surface area, and include objects like cubes, spheres, cones, and cylinders․ A comprehensive list of geometry formulas in PDF format typically includes formulas for calculating these properties, making it easier to analyze and manipulate these shapes in various applications․
Two-Dimensional Shapes
Two-dimensional shapes, often referred to as plane figures, are fundamental geometric objects that reside entirely within a two-dimensional plane․ They are characterized by their perimeter, which represents the total length of their boundary, and their area, which quantifies the amount of space they enclose․ Common examples of 2D shapes include triangles, squares, rectangles, circles, and parallelograms․ A geometry formulas PDF would likely include formulas for calculating the perimeter and area of each of these shapes, providing a handy reference for students, educators, and anyone working with geometric calculations․
Three-Dimensional Shapes
Three-dimensional shapes, also known as solid figures, exist in three-dimensional space and possess volume, surface area, and other properties that distinguish them from their two-dimensional counterparts․ These shapes are encountered in everyday life, ranging from simple objects like cubes and spheres to more complex forms found in architecture and engineering․ A geometry formulas PDF would typically include formulas for calculating the volume and surface area of various three-dimensional shapes, such as cubes, spheres, cylinders, cones, and pyramids․ These formulas enable the determination of the space occupied by these shapes and the total area of their outer surfaces, proving invaluable in fields like engineering, design, and physics․
Formulas for Area and Perimeter
A geometry formulas PDF would prominently feature formulas for calculating area and perimeter, two fundamental concepts in geometry that describe the extent of a two-dimensional shape․ Area represents the amount of surface enclosed by a shape, often measured in square units, while perimeter refers to the total length of its boundary, measured in linear units․ The PDF would typically include formulas for calculating the area and perimeter of basic shapes like rectangles, squares, triangles, and circles․ These formulas are essential for a wide range of applications, from calculating the space required for a building to determining the amount of fencing needed for a garden․ Understanding these formulas is crucial for anyone working with geometric shapes, whether in academics, engineering, or everyday life․

Rectangle/Square
A geometry formulas PDF would invariably include formulas for calculating the area and perimeter of rectangles and squares, two of the most basic and commonly encountered geometric shapes․ For a rectangle, with length ‘l’ and width ‘w’, the area is calculated as A = l * w, while the perimeter is P = 2l + 2w․ Squares, being special cases of rectangles with all sides equal, have a simplified formula for area, A = s², where ‘s’ represents the side length․ The perimeter of a square is P = 4s․ These formulas are fundamental for various applications, from calculating the area of a room to determining the length of fencing needed for a rectangular enclosure․ They are also essential for understanding more complex geometric concepts that build upon these basic shapes․
Triangle
A comprehensive geometry formulas PDF would undoubtedly include formulas for calculating the area and perimeter of triangles, a fundamental geometric shape․ The perimeter of a triangle is simply the sum of its three sides⁚ P = a + b + c, where ‘a’, ‘b’, and ‘c’ represent the lengths of the sides․ The area of a triangle can be calculated using the formula A = (1/2) * b * h, where ‘b’ is the base of the triangle and ‘h’ is its height․ However, the area can also be calculated using Heron’s formula, which relates the area to the lengths of the sides⁚ A = √(s(s-a)(s-b)(s-c)), where ‘s’ is the semi-perimeter (s = (a + b + c)/2)․ These formulas are essential for solving problems involving triangles, whether it’s finding the area of a triangular plot of land or determining the forces acting on a triangular structure․
Circle
A comprehensive geometry formulas PDF would undoubtedly include formulas for calculating the circumference and area of circles, essential geometric shapes found in various applications․ The circumference of a circle, which represents the distance around its edge, is calculated using the formula C = 2πr, where ‘r’ is the radius of the circle and ‘π’ (pi) is a mathematical constant approximately equal to 3․14159․ The area of a circle, representing the space it occupies, is calculated using the formula A = πr², where ‘r’ is the radius․ These formulas are fundamental in fields like engineering, physics, and architecture, where calculations involving circles are commonplace․ Whether determining the amount of material needed to build a circular structure or calculating the speed of a rotating object, these formulas play a crucial role in various applications․
Formulas for Volume and Surface Area
A comprehensive list of geometry formulas PDF would certainly include formulas for calculating the volume and surface area of three-dimensional shapes․ Volume, representing the amount of space a three-dimensional object occupies, is typically measured in cubic units, while surface area, representing the total area of all its surfaces, is measured in square units․ These formulas are essential for various applications, including engineering, architecture, and packaging, where understanding the properties of three-dimensional objects is crucial․ For instance, calculating the volume of a container is vital for determining its capacity, while calculating the surface area of a building is essential for estimating the amount of material needed for construction․ These formulas are crucial for solving practical problems involving three-dimensional objects, demonstrating the importance of geometric principles in various fields․
Cube
A cube, a regular hexahedron, is a three-dimensional shape with six square faces, twelve edges, and eight vertices․ Its simplicity makes it a fundamental shape in geometry, and its formulas for volume and surface area are relatively straightforward․ The volume of a cube is calculated by cubing the length of one of its sides, represented by the formula V = s³, where ‘s’ is the side length․ The surface area of a cube is calculated by multiplying the area of one of its square faces by six, as each face is congruent, resulting in the formula SA = 6s², where ‘s’ is the side length․ These formulas are essential for calculating the capacity and outer surface area of cube-shaped objects, finding applications in various fields like packaging, construction, and engineering․
Sphere
A sphere, a perfectly round three-dimensional object, is defined as the set of all points in space that are equidistant from a given point, its center․ Formulas for calculating its volume and surface area are crucial in various fields, including astronomy, engineering, and physics․ The volume of a sphere is calculated using the formula V = (4/3)πr³, where ‘r’ is the radius․ This formula demonstrates that the volume is directly proportional to the cube of the radius․ The surface area of a sphere is calculated using the formula SA = 4πr², showcasing that the surface area is proportional to the square of the radius․ These formulas are essential for understanding the properties of spherical objects and have applications in various fields, from calculating the volume of planets to determining the surface area of bubbles․
Cylinder
A cylinder is a three-dimensional geometric shape characterized by two parallel circular bases connected by a curved surface․ It is widely encountered in everyday objects, from cans to pipes, and its volume and surface area calculations are essential in various applications․ The volume of a cylinder is calculated using the formula V = πr²h, where ‘r’ represents the radius of the circular base and ‘h’ represents the height of the cylinder․ This formula reveals that the volume is directly proportional to the square of the radius and the height․ The surface area of a cylinder is calculated using the formula SA = 2πrh + 2πr², which includes the area of the two circular bases and the curved surface area․ Understanding these formulas enables efficient calculation of the capacity and outer covering of cylindrical objects, crucial in fields like engineering and design․
Trigonometry Formulas
Trigonometry, the study of triangles and their relationships, plays a crucial role in various fields, including engineering, physics, and astronomy․ Trigonometry formulas provide a framework for analyzing and solving problems involving angles, sides, and relationships within triangles․ These formulas are often expressed in terms of trigonometric functions like sine, cosine, and tangent, which relate angles to the ratios of sides in a right triangle․ Common trigonometric formulas include the Pythagorean Theorem, which establishes the relationship between the sides of a right triangle (a² + b² = c²), and the trigonometric identities, which define relationships between different trigonometric functions․ Understanding these formulas is essential for calculating distances, angles, and other geometric properties in various applications․
Right Triangle Formulas
Right triangles, with their distinctive 90-degree angle, are fundamental in trigonometry and geometry․ Right triangle formulas provide a framework for calculating various properties of these triangles, including side lengths, angles, and area․ The Pythagorean Theorem, a cornerstone of right triangle geometry, establishes the relationship between the sides of a right triangle⁚ a² + b² = c², where ‘a’ and ‘b’ represent the lengths of the legs and ‘c’ represents the length of the hypotenuse․ Trigonometric ratios, such as sine, cosine, and tangent, are used to relate angles to the ratios of sides in a right triangle․ These ratios are expressed as⁚ sin(θ) = opposite/hypotenuse, cos(θ) = adjacent/hypotenuse, and tan(θ) = opposite/adjacent․ Understanding these formulas enables the calculation of unknown sides, angles, and areas in right triangles, making them essential tools in solving various geometric problems․
Unit Circle
The unit circle, a fundamental concept in trigonometry, is a circle with a radius of 1 unit centered at the origin of a coordinate plane․ It serves as a visual representation for understanding trigonometric functions and their relationships to angles․ The unit circle allows for the determination of trigonometric values (sine, cosine, tangent, and their reciprocals) for any angle, not just acute angles within a right triangle․ By plotting points on the circle and drawing lines to the origin, the trigonometric functions can be represented as the ratios of the coordinates of these points․ The unit circle also provides a clear visualization of the periodic nature of trigonometric functions, as the values repeat in a cyclical pattern; Understanding the unit circle is crucial for solving trigonometric equations, graphing trigonometric functions, and comprehending their applications in fields like physics, engineering, and signal processing․
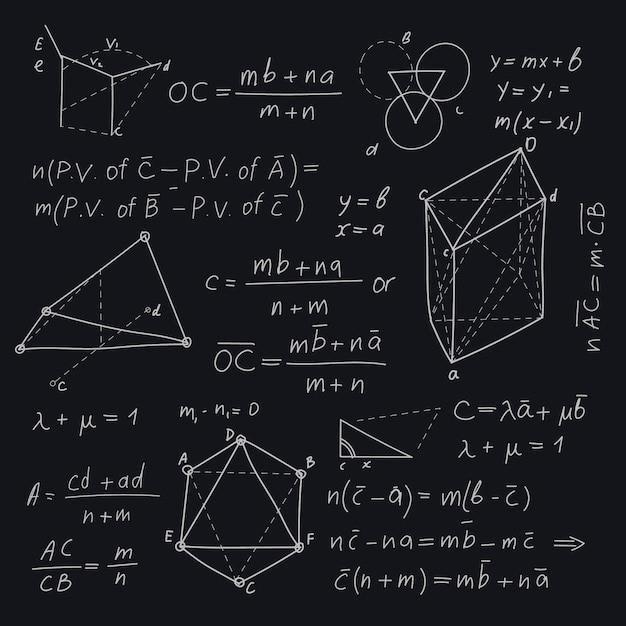
Coordinate Geometry Formulas
Coordinate geometry, a branch of geometry that utilizes a coordinate system to represent geometric shapes and figures, relies on various formulas to calculate distances, midpoints, slopes, and equations of lines․ These formulas are essential for solving problems involving lines, circles, and other geometric objects․ The distance formula, for example, calculates the distance between two points in a coordinate plane․ The midpoint formula determines the coordinates of the midpoint of a line segment․ The slope formula calculates the steepness of a line, representing its rate of change․ These formulas are widely used in various fields, including engineering, physics, and computer graphics, enabling precise calculations and analysis of geometric relationships within a coordinate system․
Distance Formula
The distance formula is a fundamental concept in coordinate geometry that calculates the distance between two points in a coordinate plane․ It is derived from the Pythagorean theorem, which relates the sides of a right triangle․ The formula expresses the distance as the square root of the sum of the squared differences of the x-coordinates and the y-coordinates of the two points․ This formula is widely used in various applications, including determining the length of a line segment, finding the distance between two objects in a coordinate system, and solving problems involving geometric figures like triangles and circles․ It allows for precise calculations of distances between points, facilitating further analysis and problem-solving in coordinate geometry․
Midpoint Formula
The midpoint formula is a simple and efficient tool for determining the coordinates of the midpoint of a line segment․ It works by averaging the x-coordinates and the y-coordinates of the two endpoints of the line segment․ The midpoint is essentially the point that divides the line segment into two equal parts․ This formula is particularly useful in various geometric applications, including finding the center of a circle, determining the location of a point that bisects a line, and solving problems involving symmetry and transformations․ Its simplicity and effectiveness make it a valuable tool for understanding and working with geometric concepts in coordinate geometry․